The Gaussian-School of Higher-Arithmetic (1831, updated 2022) ; tutorial #9.
Factoring the decryption of complex exponential numbers.
Click on the above sketch to proceed with the arithmetic required for Quantum-Relativity.
MIIS stands for Machine (electronic calculator) Induced Innumeracy Syndrome.
Author's notes:
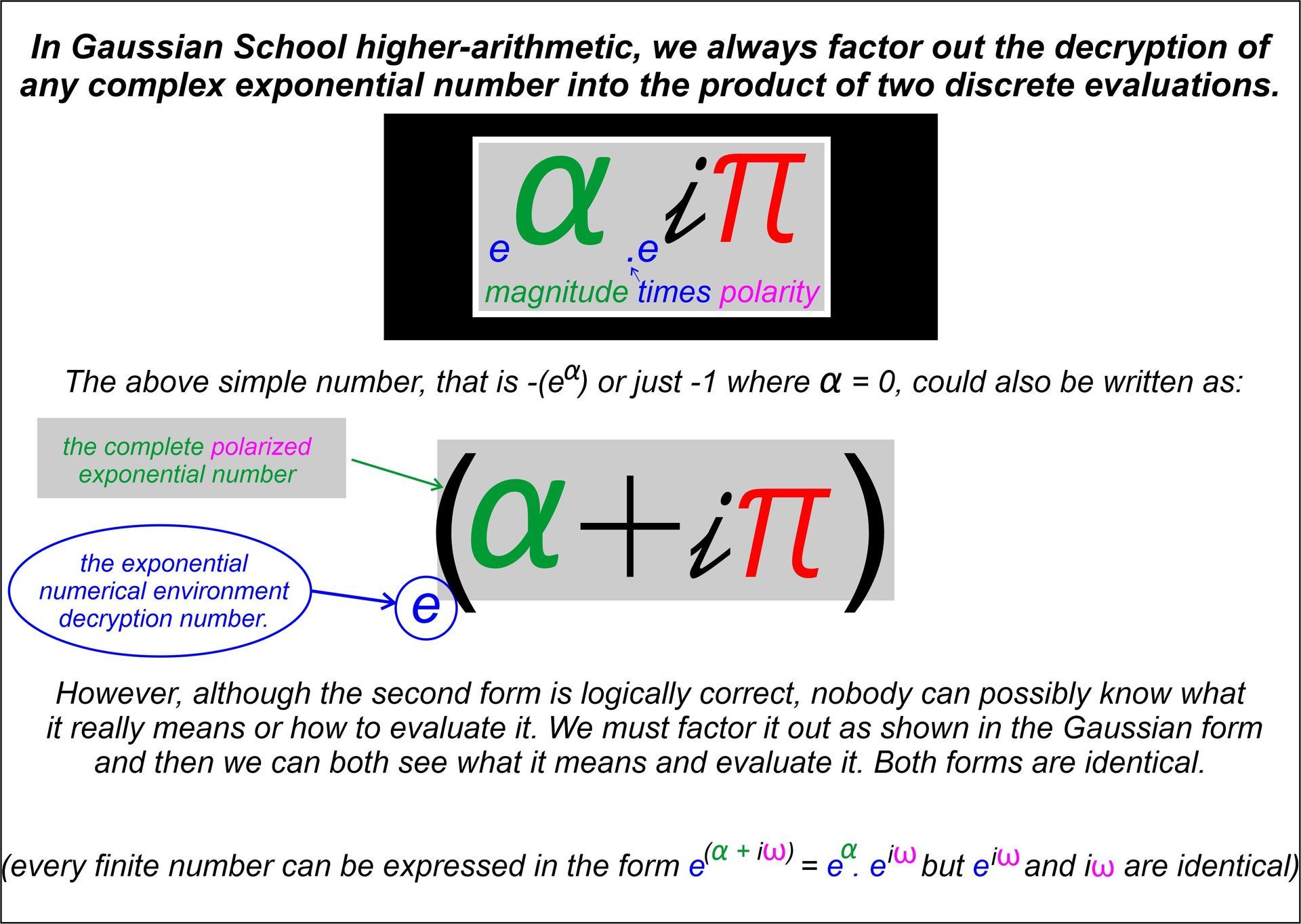
In any expression a^b
we need to think of the a
as a flat (finger counting) number and the b
as an exponential number, the expression a^b
decrypts the b
from an exponential number into a flat number, indeed a^b
is a flat number itself, we have just not calculated it yet but the answer must be a flat number. We need never decrypt b
at all, we can remain in the exponential world that b
belongs to for as long as we find that convenient. In the case of complex exponentials based on a
and expressed in the form (b + ic)
it is silly to try and decrypt that in one lump, so instead of tackling the decryption as the mind numbing a^(b + ic)
we can just factor that out as (a^b).(a^(ic))
which is highly convenient because a^(ic)
and ic
are always and everywhere identical.
However, if a is not equal to e then c will refer to a numerical polarity rotation that would not harmonise, the c would refer to a rotation with units other than perfect quadrants. We live in an exponential Universe that is based upon e and only e, so we never need theoretical unnatural solutions to hypothetical exponentials based upon any other base than e. This is because e^ic always and everywhere evaluates to simply ic and
where the c will mean a rotation of c quadrants on the flat rotational plane. While it is maybe amusing for people to calculate how things would be in a say decimal exponential universe, we as scientists, can be confident that such a hypothetical universe would not work, it would exhibit the property of harmonic chaos. We all live in an orderly Universe and need never concern ourselves with any such hypothetical unnatural exponential chaos. Over 300 years ago the irrational number e was called the natural
exponential base for a reason of course. (Or logarithmic base, but exponential and logarithmic are mathematically identical terms.)